Research Article

 

Prediction of Fortnightly Test-Day Milk Yields Using Four Different Lactation Curve Models in Indian Murrah Buffalo

 

Saroj Kumar Sahoo, Avtar Singh, Pushp Raj Shivahre, Manvendra Singh, Soumya Dash, Shakti Kant Dash

Dairy Cattle Breeding Division, National Dairy Research Institute (NDRI), Karnal, Haryana-13200, India.

 

Editor | Kuldeep Dhama, Indian Veterinary Research Institute, Uttar Pradesh, India.

Received | November 24, 2014; Revised | November 27, 2014; Accepted | November 28, 2014; Published | December 01, 2014

*Correspondence | Saroj Kumar Sahoo, National Dairy Research Institute, Karnal, Haryana-13200, India; Email: saroj6804@gmail.com

Citation | Sahoo SK, Singh A, Shivahre PR, Singh M, Dash S, Dash SK (2014). Prediction of fortnightly test-day milk yields using four different lactation curve models in Indian Murrah Buffalo.. Adv. Anim. Vet. Sci. 2 (12): 647-651.

DOI | http://dx.doi.org/10.14737/journal.aavs/2014/2.12.647.651

ISSN (Online) | 2307-8316; ISSN (Print) | 2309-3331>

Copyright © 2014 Sahoo et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

 

 

INTRODUCTION

 

Lactation curve is the graphical representation of milk yield against time (Brody et al., 1923). Dairy cattle and buffaloes with more stable lactation curve are more persistent than those which show a rapid increase in the daily milk yield from calving to peak followed by a rapid decline. Very scanty work on lactation curve has been reported in Murrah buffaloes, the best dairy buffalo breed of India. Hence, the present investigation has been proposed to fit and compare the lactation curve models for describing the shape of the lactation curve in Murrah buffaloes and prediction of fortnightly test day milk yields.

 

 

MATERIALS AND METHODS

 

Data of 18871 fortnightly test day milk yield (FTDMY) records during first lactation of 961 Murrah buffaloes calved during 1977-2012 at the National Dairy Research Institute, Karnal were collected. The climate of the farm is subtropical in nature. The lowest temperature falls to 2°C during the winter months, whereas the highest temperature goes up to 45°C during the summer. The annual rainfall is about 760 to 960mm, out of which most of the rainfall is received during the months of July and August. The relative humidity ranges from 41% to 85%.

 

A total of 21 fortnightly test day milk yield records (6th day, 20th day, 35th day,. . . , 305th day) were taken at an interval of 15days from daily milk yield recording register. The data were used to estimate lactation curve parameters of the four lactation curve models via. Gamma-type function (GF), Exponential function (EF), Mixed log function (MLF) and polynomial regression function (PRF) for developing the prediction model for estimation of fortnightly test day milk yields in Murrah buffaloes.

 

1. Gamma-type function: (Wood, 1967)

Yt = atbe-ct

In its logarithmic form, this reduces to

ln (Yt) = ln (a) + b ln (t) – ct

 

2. Exponential function (Catillo, 2002)

Yt = a+be-0.70t+ct

 

3. Mixed log function (Guo and Swalve, 1995)

Yt=a+bt0.5+clogt

In the models, Yt = average daily yield in the tth test day of lactation; a = initial milk yield just after calving; b= ascending slope parameter up to the peak yield; c=descending slope parameter and t = length of time since calving.

 

4. Polynomial regression function (Ali and Schaeffer, 1987)

Yt=a+bx+cx2+dlog (1/x)+elog (1/x)2

In this model, Yt = Average milk yield in tth week of lactation, a = Associated with peak yield, b & c = Associated with the decreasing slope, d & e = Associated with the increasing slope, x = t /305

 

 

RESULTS AND DISCUSSION

 

The least squares means of the FTDMYs were calculated for adjustment of effect of non-genetic factors (season, year of calving and age at first calving groups). The average FTDMYs increases from 3.93±0.05 kg in first test day (6th day of lactation) to a peak yield of 7.92±0.06 kg on test day 4 (50th day of lactation), and subsequently declined to 2.35±0.07 kg in last test day. In accordance with the present findings, Chakraborty et al. (2010) observed the minimum least-squares means as 4.43 ± 0.09 kg on monthly test day1 (MTD 1) (6th day) and maximum as 8.11 ± 0.25 kg on MTD 3 (65th day) in Murrah buffaloes.

 

Various lactation curve parameters (a, b, c, d and e) were estimated to fit into the mathematical models to obtain the prediction equation for FTDMYs (Table 1). The four lactation curve functions (Table 2) were developed after fitting the values of the lactation curve parameters for predicting FTDMYs.

 

 

Table 1: Estimated lactation curve parameters of different functions in Murrah buffalo

Sl. No.	Lactation curve functions	Parameters
		a	b	c	d	e
1.	GF	4.52	0.81	0.14	-	-
2.	EF	10.38	-12.68	-0.34	-	-
3.	MLF	10.97	-7.22	8.29	-	-
4.	PRF	2.31	1.61	-0.04	-4.1	-3.14

 

 

Table 2: Different lactation curve functions with parameters for prediction of FTDMY in Murrah buffaloes

Sl. No.	Function	Parameters of function	R2 (%)	RMSE (kg)
1.	GF	Yt = 4.52*t0.81e-0.14*t	93.13	0.1
2.	EF	Yt = 10.38-12.68*e-0.70*t -0.34*t	94.35	0.09
3.	MLF	Yt = 10.97-7.22*t0.5+8.29log(t)	96.96	0.06
4.	PRF	Yt= 2.31+1.61*(t/305)-0.04*(t/305)2-4.1*log(305/t)-3.14*log(305/t)2	99.78	0.02

 

 

Table 3: Predicted FTDMY and error (kg) of different lactation curve functions in Murrah buffaloes

FTDMY	Observed value(LS means)	GF		EF		MLF		PRF
		Predicted	Error	Predicted	Error	Predicted	Error	Predicted	Error
TD 1	3.93	3.94	0.01	3.75	-0.18	3.75	-0.18	3.88	-0.05
TD 2	6.49	6.02	-0.47	6.58	0.09	6.50	0.01	6.70	0.21
TD 3	7.60	7.29	-0.31	7.82	0.22	7.56	-0.04	7.50	-0.10
TD 4	7.92	8.02	0.10	8.27	0.35	8.01	0.09	7.77	-0.15
TD 5	7.90	8.38	0.48	8.32	0.42	8.16	0.26	7.84	-0.06
TD 6	7.79	8.48	0.69	8.18	0.39	8.12	0.33	7.82	0.03
TD 7	7.69	8.38	0.69	7.94	0.25	7.98	0.29	7.75	0.06
TD 8	7.59	8.15	0.56	7.65	0.06	7.77	0.18	7.63	0.04
TD 9	7.42	7.83	0.41	7.33	-0.09	7.51	0.09	7.49	0.07
TD 10	7.28	7.44	0.16	7.01	-0.27	7.21	-0.07	7.30	0.02
TD 11	7.04	7.02	-0.02	6.68	-0.36	6.88	-0.16	7.08	0.04
TD 12	6.86	6.57	-0.29	6.35	-0.51	6.54	-0.32	6.82	-0.04
TD 13	6.62	6.12	-0.50	6.01	-0.61	6.18	-0.44	6.52	-0.10
TD 14	6.09	5.67	-0.42	5.68	-0.41	5.81	-0.28	6.18	0.09
TD 15	5.77	5.24	-0.53	5.34	-0.43	5.43	-0.34	5.79	0.02
TD 16	5.40	4.82	-0.58	5.01	-0.39	5.05	-0.35	5.36	-0.04
TD 17	4.93	4.42	-0.51	4.67	-0.26	4.67	-0.26	4.87	-0.06
TD 18	4.38	4.04	-0.34	4.33	-0.05	4.28	-0.10	4.34	-0.04
TD 19	3.77	3.68	-0.09	4.00	0.23	3.88	0.11	3.75	-0.02
TD 20	3.07	3.35	0.28	3.66	0.59	3.49	0.42	3.10	0.03
TD 21	2.35	3.04	0.69	3.33	0.98	3.10	0.75	2.40	0.05

 

 

The GF gave the lowest R2 value (93.13%), with thehighest RMSE value (0.1 kg). Dimauro et al. (2005)reported similar R2 value (93.7%) in Italian water buffaloes using this function, whereas an R2 value of 96% has been reported by Kumar and Bhat (1979) and Aziz et al. (2006) in Indian buffaloes and Egyptian buffaloes, respectively. The EF gave a higher R2 value (94.35%) with a comparatively lower RMSE value (0.09 kg). Similar R2 value (94.4%) was also reported in Italian water buffaloes (Dimauro et al., 2005). The R2 and RMSE values from MLF were still better i.e. 96.96% and 0.06 kg, respectively. In accordance with this finding, an R2 value of 96.7% was reported by Kumar (2007) in Murrah buffalo. The PRF gave the highest R2 value (99.78%) and the lowest RMSE value (0.02 kg) for Murrah buffaloes in the present study. In Italian water buffaloes, Dimauro et al. (2005) reported R2-value of 96.7% using this function.

 

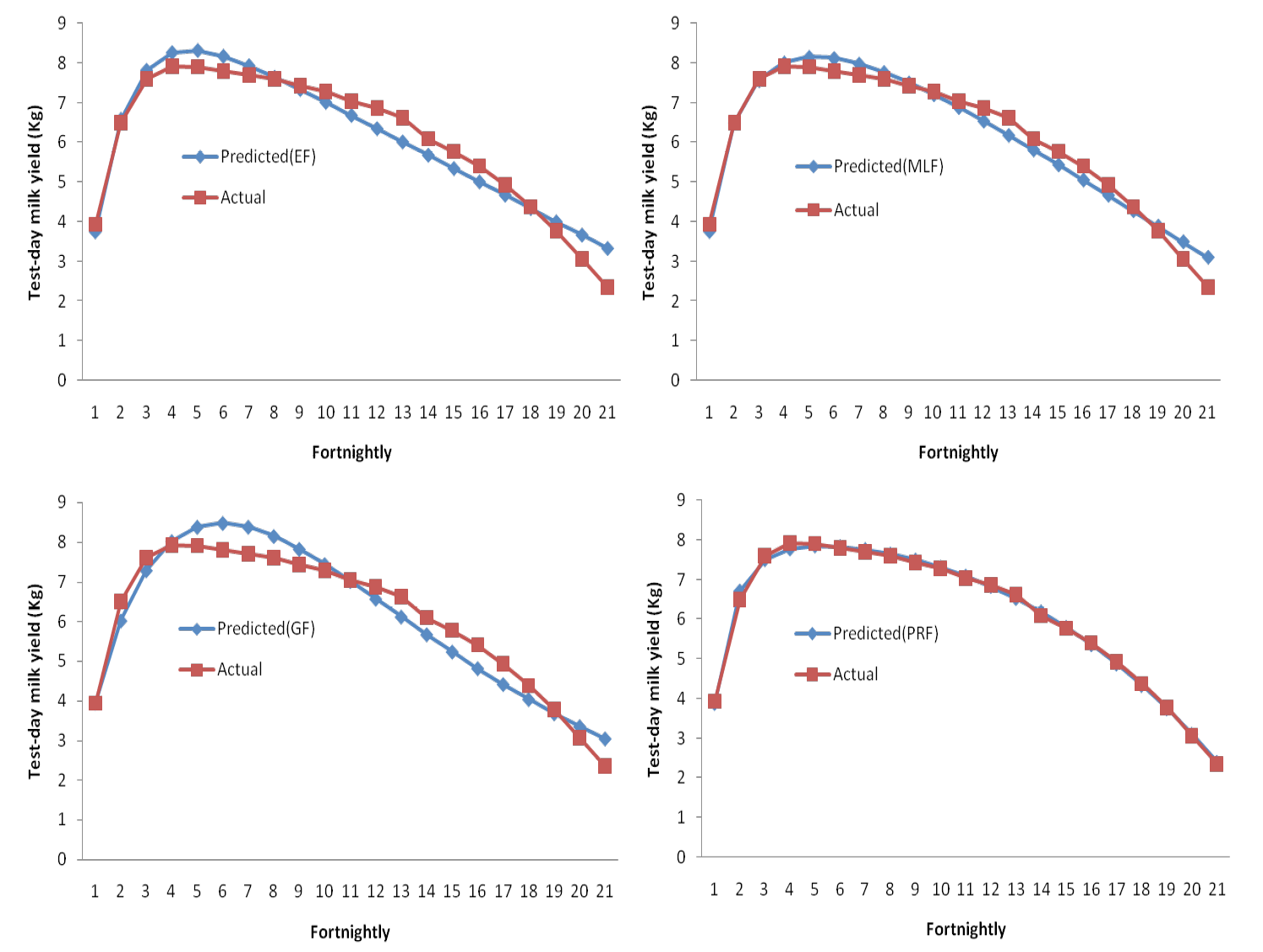
PRF which explained highest coefficient of determination value with lowest root mean squares error predicted the peak fortnightly test day milk yield to be 7.84 kg in the 5th test day of lactation. EF and MLF also predicted the peak yield as 8.32 kg and 8.16 kg in 5th test day of lactation, respectively. However, by GF the peak yield was found to be 8.48 kg in 6th test day of lactation. In Italian water buffaloes using GF, EF and PRF, Dimauro et al. (2005) reported peak yield of 10.9kg, 11.3kg and 11.5kg in 33rd, 26th and 29th day of lactation, respectively. The predicted and actual fortnightly test day milk yield records of different functions have been presented in table 3.

 

The overall observed and predicted FTDMYs for all 4 lactation curve functions were plotted graphically (Figure 1). The closeness of PRF was almost of similar order of magnitude as the observed one. This may be due to the fact that this function accounted for rising and declining segments of the lactation curve.

 

 

Conclusions

 

In the present investigation it was found that on an average, the peak yield from all lactation curves functions was found to be highest around 65th day of lactation in Murrah buffaloes. PRF is the best function among four models studied followed by mixed log function explaining highest coefficient of determination with lowest root mean square error and therefore these two functions are recommended for prediction of FTDMYs in Murrah buffaloes.

 

 

Acknowledgements

 

We sincerely thank the Director, National Dairy Research Institute, Karnal and Head, Dairy Cattle Breeding Division for providing necessary facilities for conducting this study. Financial support in form of DST-INSPIRE fellowship (Govt. of India) is duly acknowledged.

 

 

Conflict of Interest

 

The authors declare that there is no conflict of interest.

 

 

References

 

Ali TE, Schaeffer LR (1987). Accounting for covariances among test day milk yields in dairy cows. Can. J. Anim. Sci. 67(3): 637-644. http://dx.doi.org/10.4141/cjas87-067

 

Aziz MA, Shalaby NA, El-Shafie OM, Mahdy AT, Nishida A (2006). Comparison between the shapes of lactation curve of Egyptian buffalo milk yield estimated by the incomplete gamma function and a new model. Livest. Res. Rural Dev. 18(5): Article # 59.

 

Brody S, Ragsdale AC, Turner CW (1923). The rate of decline of milk secretion with the advance of the period of lactation. J. Gen. Physiol. 5(4): 441-444. http://dx.doi.org/10.1085/jgp.5.4.441

 

Catillo G, Macciotta NPP, Carretta A, Cappio Borlino A (2002). Effects of age and calving eeason on lactation curves of milk production traits in Italian water buffaloes. J. Dairy Sci. 85 (5):1298-1306. http://dx.doi.org/10.3168/jds.S0022-0302(02)74194-5

 

Chakraborty D, Dhaka SS, Pander BL, Yadav AS, Singh S, Malik PK (2010). Prediction of lactation milk yield from test day records in Murrah buffaloes. Indian J. Anim. Sci. 80(3): 244-245.

 

Dimauro C, Catillo G, Bacciu N, Macciotta NPP (2005). Fit of different linear models to the lactation curve of Italian water buffalo. Ital. J. Anim. Sci.4(2): 22-24.

 

Guo Z, Swalve HH (1995). Modelling of the lactation curve as a sub-model in the evaluation of test day records. Proc. Interbull Mtg. Prague, Uppsala, Interbull Bull. No. 11.

 

Kumar R, Bhat PN (1979). Lactation curves in Indian buffaloes. Indian J. Dairy Sci. 32(2): 156-160.

 

Kumar S (2007). A comparative study of lactation curve models for prediction of lactation milk yield in Murrah buffaloes. M.V.Sc. Thesis, NDRI, (Deemed University), Karnal, India.

 

Wood PDP (1967). Algebraic model of lactation curve in cattle. Nature. 216(5111): 164-165. http://dx.doi.org/10.1038/216164a0